Answer:

and we can set up the following equation
tex]z=-1.282<\frac{a-104.5}{23.62}[/tex]
And if we solve for a we got

And the best answer for this case would be:
b) $74.23
Explanation:
Let X the random variable that represent the stocks price of a population, and for this case we know the distribution for X is given by:

Where
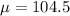 and
and
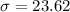
For this part we want to find a value a, such that we satisfy this condition:
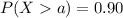 (a)
(a)
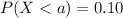 (b)
(b)
As we can see on the figure attached the z value that satisfy the condition with 0.10 of the area on the left and 0.90 of the area on the right it's z=-1.282. On this case P(Z<-1.282)=0.10 and P(z>-1.282)=0.90
If we use condition (b) from previous we have this:
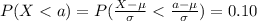

and we can set up the following equation
tex]z=-1.282<\frac{a-104.5}{23.62}[/tex]
And if we solve for a we got

And the best answer for this case would be:
b) $74.23