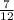Answer:
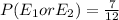
Explanation:
Given
Two cubes of side 1 - 6
Required
Probability that the outcome of the roll is an odd sum or a sum that is a multiple of 5
First, the sample space needs to be listed;
Let
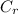 represent the Red cube
represent the Red cube
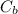 represent the Blue cube
represent the Blue cube
S represent the sample space
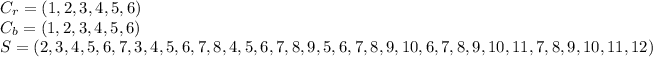 S is gotten by getting the sum of
S is gotten by getting the sum of
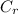 and
and
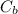
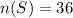
Calculating the Probability
Let
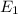 represent the event that an outcome is an odd sum
represent the event that an outcome is an odd sum
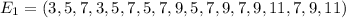
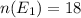
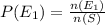
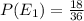
Let
 represent the event that an outcome is a multiple of 5
represent the event that an outcome is a multiple of 5
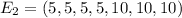
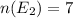
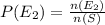
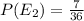
Let
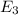 represent the event that an outcome is an odd sum and a multiple of 5
represent the event that an outcome is an odd sum and a multiple of 5
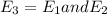

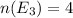
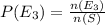
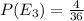
Calculating
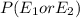

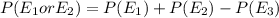
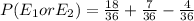
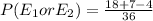
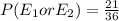
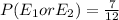
Hence, the probability that the outcome of the roll is an odd sum or a sum that is a multiple of 5 is