Question

A laptop producing company also produces laptop batteries, and claims that the batteries

it produces power a laptop for about 4:00 hours. But, you doubted the claim and collected
data from 500 laptop users of the same brand and battery, and you found out the battery
powers the laptop for about 3:00 hours and 30 minutes. Considering an alpha of 0.05,
prove the claim of the company is true or false or show whether you accept the
company’s claim or reject it? Please also write H0 and Ha statements for testing your
hypothesis

Answer 1

There is enough evidence to support the claim that the batteries power the laptops for significantly less than 4 hours. (P-value = 0).

The null and alternative hypothesis are:

`H_0: \mu=4\\\\H_a:\mu< 4`

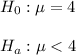
Explanation:

The question is incomplete: To test this claim a sample or population standard deviation is needed.

We will estimate that the sample standard deviation is 2 hours, and use a t-test to test that claim.

NOTE (after solving): The difference between the sample mean and the mean of the null hypothesis is big enough to reject the null hypothesis, even when we have a sample standard deviation of 3.5 hours, which can be considered bigger than the maximum standard deviation for the sample.

This is a hypothesis test for the population mean.

The claim is that the batteries power the laptops for significantly less than 4 hours.

Then, the null and alternative hypothesis are:

`H_0: \mu=4\\\\H_a:\mu< 4`

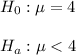
The significance level is 0.05.

The sample has a size n=500.

The sample mean is M=3.5.

As the standard deviation of the population is not known, we estimate it with the sample standard deviation, that has a value of s=2.

The estimated standard error of the mean is computed using the formula:

`s_M=(s)/(√(n))=(2)/(√(500))=0.0894`

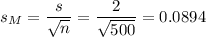
Then, we can calculate the t-statistic as:

`t=(M-\mu)/(s/√(n))=(3.5-4)/(0.0894)=(-0.5)/(0.0894)=-5.5902`

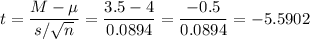
The degrees of freedom for this sample size are:

`df=n-1=500-1=499`

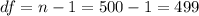
This test is a left-tailed test, with 499 degrees of freedom and t=-5.5902, so the P-value for this test is calculated as (using a t-table):

`P-value=P(t<-5.5902)=0`

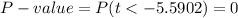
As the P-value (0) is smaller than the significance level (0.05), the effect is significant.

The null hypothesis is rejected.

There is enough evidence to support the claim that the batteries power the laptops for significantly less than 4 hours.