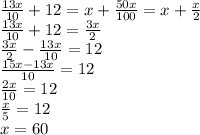Question

Lesson 9: Problem Solving When the Percent Changes

Exit Ticket
Tamia and Laniece were selling magazines for a charity. In the
first week, Tamia sold 30% more than Laniece. In the second
week, Tamia sold 12 magazines, but Laniece did not sell any. If
Tamia sold 50% more than Laniece by the end of the second
week, how many magazines did Laniece sell? Choose any
model to solve the problem. Show your work to justify your
answer.​

Answer 1

Laniece had 60 magazines

Explanation:

Given: In the first week, Tamia sold 30% more than Laniece. In the second week, Tamia sold 12 magazines, but Laniece did not sell any. Tamia sold 50% more than Laniece by the end of the second week

To find: Number of magazines sold by Laniece

Solution:

Let number of magazines sold by Laniece in the first week be x.

Number of magazines sold by Tamia in the first week =
`x+(30)/(100) x=(130x)/(100) =(13x)/(10)`

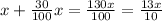
Number of magazines sold by Tamia in the second week = 12

Total number of magazines sold by Tamia at the end of the second week =
`(13x)/(10)+12`

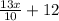
Total number of magazines sold by Laniece at the end of the second week = x

According to question,

`(13x)/(10)+12=x+(50x)/(100)=x+(x)/(2)\\(13x)/(10)+12=(3x)/(2)\\(3x)/(2)-(13x)/(10) =12\\(15x-13x)/(10)=12\\(2x)/(10)=12\\(x)/(5)=12\\x=60`