Answer:
Ah! Thank you for a little challenge!
Explanation:
To do this, we need to first find our new lengths!
Height=

Radius=
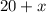
Now, to find the Volume!
Using the volume formula for a cylinder(v=pi*r^2*h)
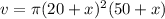
So, we have our equation! let's solve!
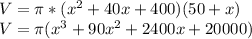
Now, I'm guessing you had trouble with it because it looked really scary.
So what I did in the first step was expand (20+x)^2, and I used the special formula(forgot what else it was called, (a+b)^2=a^2+2ab+b^2)
Next, I multiplied the expand form of that with (50+x), and the way to do that is by multiplying each term in the parentheses with every term in the next parentheses(distributive property).
So, uh, that's it! Not very scary, just a bit of in-head math!
Hope this helps! If you want to learn more or need more help, check out Khan Academy for the "squares of binomials" or "polynomials"