Question

Over several years, students of Professor Robin Lock have flipped a large number of coins and recorded whether the flip landed on heads or tails. As reported in a 2002 issue of Chance News, these students has observed 14,709 heads in a total of 29,015 flips.

Required:
a. What are the observational units for this study?
b. Determine the theory-based p-value for testing whether the long-run proportion of heads differs from 0.50.
c. What conclusion would you draw at the 0.05 significance level?
d. Are your results practically important? Why/why not?

Answer 1

a) The observational units for this study are coins

b) The test is a two-tailed test.

c) Since the p-value is < α, we can therefore conclude that long-run proportion of heads significantly differ from 0.50

d) The findings are known as statistically important, due to the very large sample size. But the difference between the 0.50 ratio hypothesized and the 0.51 ratio observed isn't very large. Consequently , the findings are not relevant

Explanation:

a) The observational units for this study are coins

b) Number of heads = x = 14709

Number of flips = n = 29015

Sample proportion =
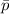`\bar{p}` = x ÷ n = 14709 ÷ 29015=0.51

H
`_(o)` : p =0.50

H
`_(a):` p
`\\e` 0.50

z =
`(\bar{p}-p)/√(p(1-p)/n) = (0.51-0.50)/√(0.50 * (1-0.50)/29015)`

z = 2.366

Using the normal distribution table, area under the normal curve to the right of z;

z = 2.366 = 0.009

The test is a two-tailed test.

Hence, p-value =
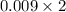`0.009 * 2` = 0.018

c) α = 0.05

p-value < α = 0.05, we reject the null hypothesis.

We can therefore conclude that long-run proportion of heads significantly differ from 0.50

d) The findings are known as statistically important, due to the very large sample size. But the difference between the 0.50 ratio hypothesized and the 0.51 ratio observed isn't very large. Consequently , the findings are not relevant