The volume of the rectangular prism is
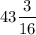 cubic meters.
cubic meters.
The volume of a rectangular prism is the product of the length, width and height of the prism. From the image, we can find that the lengths of the sides are
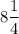 m, 6 m and
m, 6 m and
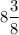 m.
m.
To find the volume, we just multiply these side lengths together.
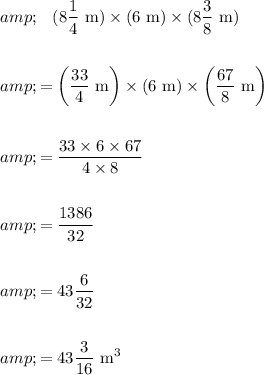
Therefore, the volume of the rectangular prism is
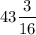 cubic meters.
cubic meters.