Question

When 893893 male workers were asked how many hours they worked in the previous​ week, the mean was 45.645.6 with a standard deviation of 14.614.6. Does this suggest that the population mean work week for men exceeds 4040 ​hours? Answer by completing parts​ (a) through​ (d).

Answer 1

a) A. The relevant variable is the population mean work week (in hours) for workers aged 18-25.

b) Null hypothesis:
`\mu \leq 40`

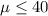
Alternative hypothesis:
`\mu > 40`

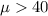
c)
`t=(45.6-40)/((14.6)/(√(893)))=11.46`

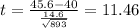
The p value for this case would be:

`p_v = P(t_(110) >11.46) \approx =0`

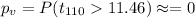
d) Since the p value is a very low value we have enough evidence to reject the null hypothesis and we can conclude that the true mean for this case exceeds 40 hours.

Explanation:

Information provided

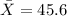`\bar X=45.6` represent the sample mean

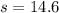`s=14.6` represent the sample standard deviation

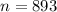`n=893` sample size

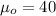`\mu_o =40` represent the value to verify

t would represent the statistic

`p_v` represent the p value

a. Identify the relevant and parameter variable. Choose the correct relevant variable below.

A. The relevant variable is the population mean work week (in hours) for workers aged 18-25.

b. State the null and alternative hypotheses. State the null hypothesis.

We want to verify if the population mean is higher than 40, the system of hypothesis would be:

Null hypothesis:
`\mu \leq 40`

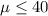
Alternative hypothesis:
`\mu > 40`

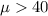
c. Calculate the statistic

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

Replacing the info given we got:

`t=(45.6-40)/((14.6)/(√(893)))=11.46`

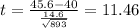
The p value for this case would be:

`p_v = P(t_(110) >11.46) \approx =0`

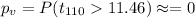
d. Conclusion

Since the p value is a very low value we have enough evidence to reject the null hypothesis and we can conclude that the true mean for this case exceeds 40 hours.