Answer:
Explanation:
The area of the square garden=

Area of a Square of Side length s
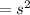
Therefore:

Perimeter
Perimeter of a Square of Side length s=4s
Therefore, the perimeter of the garden =4*16.34=65.36 ft
Since 65 ft < 65.36 ft
Therefore, if you had 65 ft of fencing, it will not be enough to go around and cover the garden.