Answer:
-The measure of
 :
:
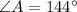
-The measure of
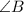 (if needed):
(if needed):
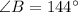
Explanation:
-Set the measure of
 on one side and set the measure of
on one side and set the measure of
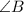 on the other side and it will be written as:
on the other side and it will be written as:

-Then, solve for
 :
:

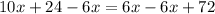



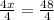
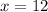
-After you have found the value of
 , plug in both the measure of
, plug in both the measure of
 and
and
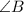 in order to get the actual angle measurement of
in order to get the actual angle measurement of
 and
and
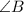 :
:
Finding the measure of
 :
:
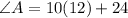
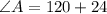
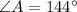
Finding the measure of
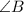 :
:
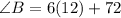
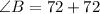
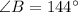
-Notice: That reason why both
 and
and
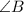 have the same measurements, its because they are Alternate Exterior Angles, based on the diagram shown.
have the same measurements, its because they are Alternate Exterior Angles, based on the diagram shown.