Answer:
1) The volume of the model is 3159 in.³
2) The height of the real facility is 61.94 ft
Explanation:
1) The parameters given are;
Volume of the Estabrook hall = 117 ft³
Scale of model = 1:4
For the scale factor of a volume, we have;
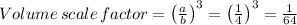
That is the volume of the model is 1/64 times the volume of the actual Estabrook hall restrooms or
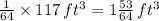
∴ The volume of the model = 1.83 ft.³
1 ft.³ = 1728 in.³
∴ 1.83 ft.³ = 1.83 ft.³ × 1728 in.³/ft.³ = 3159 in.³
2) Where the model of the floor has an area of 117 in.²
Therefore, since the volume of a rectangular prism shape = Floor area × Height, we have
The height, h = Volume/(floor area) = 3159/117 = 185.8 in.
From a scale of 1:4, we have that the height of the real facility = 4 × 185.8 in.
Hence, the height of the real facility = 743.3 in = 61.94 ft.