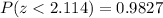Answer:


And we want to find this probability:

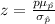
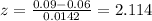
And we can find this probability:
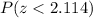
And using the normal table or excel we got:
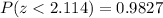
Explanation:
For this case we have the following info given:
 represent the sample size
represent the sample size
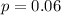 represent the true proportion
represent the true proportion
The sample proportion can be approximated with this distribution:
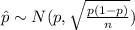
The mean is given by:

And the deviation is given by:

And we want to find this probability:

And we can use the z score formula given by:
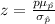
And replacing we got:
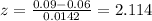
And we can find this probability:
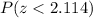
And using the normal table or excel we got: