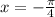Question

Tan^(2)x-tanx=0
Pls Help

Answer 1

`x=(3\pi )/(4) ,(7\pi )/(4)`

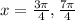
If wrong then
`x=-(\pi )/(4).`

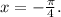
Explanation:

`tan^2(x)-tan(x)=0`

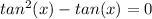
Factor out the tan(x).

`tan(x)*(tan(x)-1)=0`

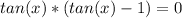
Solve for x.

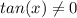`tan(x)\\eq 0\\` thus only option is tan(x)-1=0

`tan(x)-1=0\\tan(x)=1\\`

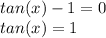
x=tan^-1(1)⇒

Either it is Quadrant 2 or 4.

`x=(3\pi )/(4) ,(7\pi )/(4)`

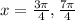
If the teacher says its wrong. Then that could only mean that its range of tan(x) is between
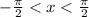`-(\pi )/(2) < x < (\pi )/(2)` thus it's only on quadrant 4.
`x=-(\pi )/(4)`