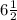Question

Solve. Enter your answer as a mixed number. The driving distance from the sports arena to Kristina's house is 11 7 10 miles. The distance from the sports arena to Luke's house is 5 2 10 miles. How much greater is the driving distance between the sports arena and Kristina's house than between the sports arena and Luke's house? The driving distance between the sports arena and Kristina's house is miles greater than between the sports arena and Luke's house.

Answer 1

Answer:
`6(1)/(2) miles`

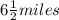
Explanation:

From the sports arena to Kristina's house =
`11(7)/(10)miles`

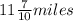
From the sports arena to Luke's house =
`5(2)/(10) miles`

Subtract Luke from Kristina

`5(2)/(10)-11(7)/(10)`

There's a confusing but easy way to solve this. However, I'm going to convert into improper fractions to make it easier.

To convert to improper fraction, take the whole number, multiply it by the denominator and add it to the numerator. All over the same denominator.

`(5*10+2)/(10) -(11*10+7)/(10)`

`(50+2)/(10)-(110+7)/(10)\\(52)/(10)-(117)/(10)`

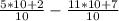
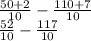
Subtract them.

`(52-117)/(10)`

`-(65)/(10)`

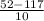
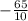
Convert to mixed number by dividing.

65/10=6

60

----

5

The 6 is the whole number, 5 the numerator and 10 the denominator. Remember the negative sign that we had.

`6(5)/(10)`

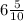
5 and 10 can be simplified

5/5=1

10/5=2

Our final answer would be:

`6(1)/(2)`