Rewriting
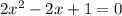 equation we get
equation we get
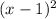 =0.
=0.
Given the quadratic equation
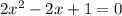
the process of completing the square involves manipulating the equation to a form that expresses a perfect square trinomial.
First, move the constant term to the right-hand side, resulting in
 −2x−1=0.
−2x−1=0.
Next, halve the coefficient of x, yielding
−2/2=−1
Squaring this result
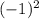 gives 1.
gives 1.
Add this value to both sides of the equation:
=
 −2x +
−2x +
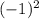
= −1 +
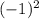
leading to
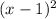 =0
=0
Upon simplification,
x−1 squared equals zero.
Therefore, the equation rewritten by completing the square is
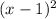 =0, indicating that the solution involves a perfect square binomial with a value of zero.
=0, indicating that the solution involves a perfect square binomial with a value of zero.