Question

The function f (t) =-16t^2 = 576 represents the height of a freely falling ballast bag that starts from rest on a ballon 576 feet above the ground. After how many seconds t does the ballast bag hit the ground.

Answer 1

The ballast bag hits the ground after 6 seconds.

Explanation:

Suppose there was a small typing mistake.

The height of the ball, after t seconds, is given by the following equation:

`f(t) = -16t^(2) + 576`

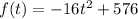
After how many seconds t does the ballast bag hit the ground.

This is t for which f(t) = 0. So

`f(t) = -16t^(2) + 576`

`-16t^(2) + 576 = 0`

`16t^(2) = 576`

`t^(2) = (576)/(16)`

`t^(2) = 36`

`t = \pm √(36)`

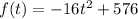
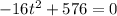
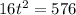
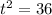
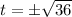
Time is a positive measure, so

`t = 6`

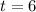
The ballast bag hits the ground after 6 seconds.