Question

How many milliliters of water are needed to prepare a 3.5M solution of NaOH if you have .5mol of the solute

Answer 1

1335.12 mL of H2O

Step-by-step explanation:

To calculate the mililiters of water that the solution needs, it is necessary to know that the volume of the solution is equal to the volume of the solute (NaOH) plus the volume of the solvent (H2O).

From the molarity formula we can first calculate the volume of the solution:

`M=(solute moles)/(solution volume)`

`Solutionvolume=(solute moles)/(M) =(5mol)/(3.5(mol)/(L) ) =1.429L`

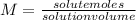
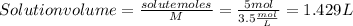
The volume of the solution as we said previously is:

Solution volume = solute volume + solvent volume

To determine the volume of the solute we first obtain the grams of NaOH through the molecular weight formula:

`MW=(mass)/(mol)`

`Mass=MW*mol=39.997(g)/(mol) *5mol=199.985g`

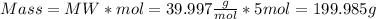
Now with the density of NaOH the milliliters of solute can be determined:

`d=(mass)/(volume)`

`Volume=(mass)/(d) =(199.985g)/(2.13(g)/(mL) ) =93.88mL of NaOH`

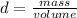
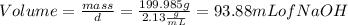
Having the volume of the solution and the volume of the solute, the volume of the solvent H2O can be calculated:

Solvent volume = solution volume - solute volume

Solvent volume = 1429 mL - 93.88 mL = 1335.12 mL of H2O