Question

In a previous​ poll, 40​% of adults with children under the age of 18 reported that their family ate dinner together seven nights a week. Suppose​ that, in a more recent​ poll, 456 of 1194 adults with children under the age of 18 reported that their family ate dinner together seven nights a week. Is there sufficient evidence that the proportion of families with children under the age of 18 who eat dinner together seven nights a week has​ decreased? Use the alpha equals 0.01 significance level.

Answer 1

We conclude that the proportion of families with children under the age of 18 who eat dinner together seven nights a week has​ increased or remained same.

Explanation:

We are given that in a previous​ poll, 40​% of adults with children under the age of 18 reported that their family ate dinner together seven nights a week.

Suppose​ that, in a more recent​ poll, 456 of 1194 adults with children under the age of 18 reported that their family ate dinner together seven nights a week.

Let p = proportion of families with children under the age of 18 who eat dinner together seven nights a week.

SO, Null Hypothesis,
`H_0` : p
`\geq` 40% {means that the proportion of families with children under the age of 18 who eat dinner together seven nights a week has​ increased or remained same}

Alternate Hypothesis,
`H_A` : p < 40% {means that the proportion of families with children under the age of 18 who eat dinner together seven nights a week has​ decreased}

The test statistics that would be used here One-sample z test for proportions;

T.S. =
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }` ~ N(0,1)

where,
`\hat p` = sample proportion of families with children under the age of 18 who eat dinner together seven nights a week =
`(456)/(1194)` = 0.38

n = sample of adults = 1194

So, the test statistics =
`\frac{0.38-0.40}{\sqrt{(0.38(1-0.38))/(1194) } }`

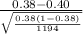
= -1.424

The value of z test statistics is -1.424.

Now, at 0.01 significance level the z table gives critical value of -2.326 for left-tailed test.

Since our test statistic is more than the critical value of z as -1.424 > -2.326, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region due to which we fail to reject our null hypothesis.

Therefore, we conclude that the proportion of families with children under the age of 18 who eat dinner together seven nights a week has​ increased or remained same.