Answer:
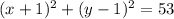
Explanation:
The standard form for the equation of a circle is
 , where h is the x coordinate of the center, k is the y coordinate, and r is the radius. To find the radius of this circle, we can simply find the distance from the center to any of the points on its circumference. Using the distance formula, you know that the distance from (-1,1) to (1,-6) is:
, where h is the x coordinate of the center, k is the y coordinate, and r is the radius. To find the radius of this circle, we can simply find the distance from the center to any of the points on its circumference. Using the distance formula, you know that the distance from (-1,1) to (1,-6) is:
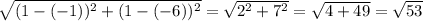
Therefore, the equation of this circle is:
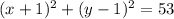
Hope this helps!