Question

At a competition with 6 runners, medals are awarded for first, second, and

third places. Each of the 3 medals is different. How many ways are there to

award the medals?

Decide if this is a permutation or a combination, and find the number of ways

to award the medals.

O

A. Permutation; number of ways = 20

O

O

B. Combination; number of ways = 20

C. Permutation; number of ways = 120

O

D. Combination; number of ways = 120

SUBMIT

Answer 1

C. Permutation; number of ways = 120

Explanation:

The order in which the runners finish is important. For example, A,B,C means that A will be awarded the 1st place medal, B the 2nd and C the 3rd. B,A,C means that B is awarded the 1st place medal, A the 2nd and C the 3rd. So we use the permutations formula to solve this question. That is, this is a permutation.

Permutations formula:

The number of possible permutations of x elements from a set of n elements is given by the following formula:

`P_((n,x)) = (n!)/((n-x)!)`

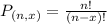
In this question:

3 medals to 6 runners. So

`P_((6,3)) = (6!)/((6-3)!) = 120`

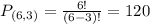
So the correct answer is:

C. Permutation; number of ways = 120