Image of the circle is attached.
Answer:
Measure of angle G = 36°
Explanation:
From the question, we are told the arc GF has a measure of 108°, i.e
m∠GF = 108°
From the circle, we can see a part of the circumference of the circle is the arc length (curve from point G to point F).
The arc length can be said to be the measure of the distance between two points along the section of a curved line which makes up an arc.
The arc length is expressed as:
A = r x Θ
Where,
A = length of arc
r = radius
Θ = arc
The measure of the central angle intercepting an arc is equal to the degree measure of the arc.
Here, the degree measure of the arc is 108° since it is equal to the central angle, E, the measure of angle E is 108°. ie m∠E = 108°
We know the total sum of angles of a triangle is 180°.
Therefore,
m∠E + m∠F + m∠G = 180°
108° + m∠F + m∠G = 180°
m∠F + m∠G = 180° - 108°
m∠F + m∠G = 72°
From the diagram we could see that F and G are parallel to each other, which means they have equal angles. Therefore, m∠F = m∠G.
Since they are equal,
m∠G =
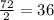
m∠G = 36°
Measure of angle G = 36°