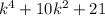Answer:
The area of the rectangle in polynomial form is
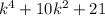
Explanation:
Given
Shape: Rectangle
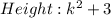

Required
Area of the rectangle
The area of a rectangle is calculated as thus;
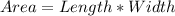
By substituting
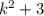 for height and
for height and
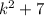 for width; This gives
for width; This gives
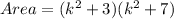
Expand the bracket
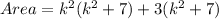
Open each bracket
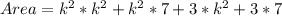
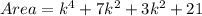
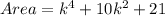
Hence, the area of the rectangle in polynomial form is