Question

Tompkins Associates reports that the mean clear height for a Class A warehouse in the United States is 22 feet. Suppose clear heights are normally distributed and that the standard deviation is 4 feet. A Class A warehouse in the United States is randomly selected. (a) What is the probability that the clear height is greater than 16 feet? (b) What is the probability that the clear height is less than 14 feet? (c) What is the probability that the clear height is between 25 and 30 feet?

Answer 1

Given Information:

Mean clear height = μ = 22 feet

Standard deviation of clear height = σ = 4 feet

Required Information:

a) P(X > 16) = ?

b) P(X < 14) = ?

c) P(25 < X < 30) = ?

Answer:

a) P(X > 16) = 93.12%

b) P(X < 14) = 2.27%

c) P(25 < X < 30) = 20.39%

Explanation:

What is Normal Distribution?

We are given a Normal Distribution, which is a continuous probability distribution and is symmetrical around the mean. The shape of this distribution is like a bell curve and most of the data is clustered around the mean. The area under this bell shaped curve represents the probability.

a) We want to find out the probability that the clear height is greater than 16 feet.

`P(X > 16) = 1 - P(X < 16)\\\\P(X > 16) = 1 - P(Z < (x - \mu)/(\sigma) )\\\\P(X > 16) = 1 - P(Z < (16 - 22)/(4) )\\\\P(X > 16) = 1 - P(Z < (-6)/(4) )\\\\P(X > 16) = 1 - P(Z < -1.5)\\\\`

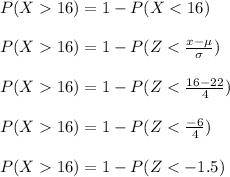
The z-score corresponding to -1.5 is 0.0668

`P(X > 16) = 1 - 0.0668\\\\P(X > 16) = 0.9312\\\\P(X > 16) = 93.12 \%`

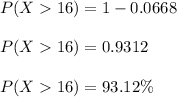
Therefore, the probability that the clear height is greater than 16 feet is 93.12%

b) We want to find out the probability that the clear height is less than 14 feet

`P(X < 14) = P(Z < (x - \mu)/(\sigma) )\\\\P(X < 14) = P(Z < (14 - 22)/(4) )\\\\P(X < 14) = P(Z < (-8)/(4) )\\\\P(X < 14) = P(Z < -2)\\\\`

The z-score corresponding to -2 is 0.02275

`P(X < 14) = 0.02275\\\\P(X < 14) = 2.27 \%`

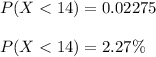
Therefore, the probability that the clear height is less than 14 feet is 2.27%

c) We want to find out the probability that the clear height is between 25 and 30 feet.

`P(25 < X < 30) = P( (x - \mu)/(\sigma) < Z < (x - \mu)/(\sigma) )\\\\P(25 < X < 30) = P( (25 - 22)/(4) < Z < (30 - 22)/(4) )\\\\P(25 < X < 30) = P( (3)/(4) < Z < (8)/(4) )\\\\P(25 < X < 30) = P( 0.75 < Z < 2 )\\\\`

The z-score corresponding to 0.75 is 0.7734 and 2 is 0.9773

`P(25 < X < 30) = P( Z < 2 ) - P( Z < 0.75 ) \\\\P(25 < X < 30) = 0.9773 - 0.7734 \\\\P(25 < X < 30) = 0.2039\\\\P(25 < X < 30) = 20.39 \%`

Therefore, the probability that a child spends more than 4 hours and less than 8 hours per day unsupervised is 20.39%

How to use z-table?

Step 1:

In the z-table, find the two-digit number on the left side corresponding to your z-score. (e.g 1.5, 2.2, 0.5 etc.)

Step 2:

Then look up at the top of z-table to find the remaining decimal point in the range of 0.00 to 0.09. (e.g. if you are looking for 1.50 then go for 0.00 column)

Step 3:

Finally, find the corresponding probability from the z-table at the intersection of step 1 and step 2.