Question

Deluxe River Cruises operates a fleet of river vessels. The fleet has two types of vessels: A type-A vessel has 60 deluxe cabins and 160 standard cabins, whereas a type-B vessel has 80 deluxe cabins and 120 standard cabins. Under a charter agreement with Odyssey Travel Agency, Deluxe River Cruises is to provide Odyssey with a minimum of 360 deluxe and 680 standard cabins for their 15-day cruise in May. It costs $42,000 to operate a type-A vessel and $51,000 to operate a type-B vessel for that period. How many of each type of vessel (x type-A and y type-B) should be used in order to keep the operating costs to a minimum

Answer 1

Answer: It should be used 2 for type-A and 3 for type-B to minimize the cost.

Explanation: As it is stipulated, x relates to type-A and y to type-B.

Type-A has 60 deluxe cabins and B has 80. It is needed a minimum of 360 deluxe cabins, so:

60x + 80y ≤ 360

For the standard cabin, there are in A 160 and in B 120. The need is for 680, so:

160x + 120y ≤ 680

To calculate how many of each type you need:

60x + 80y ≤ 360

160x + 120y ≤ 680

Isolating x from the first equation:

x =
`(360 - 80y)/(60)`

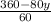
Substituing x into the second equation:

160(
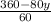`(360 - 80y)/(60)`) + 120y = 680

-3200y+1800y = 10200 - 14400

1400y = 4200

y = 3

With y, find x:

x =
`(360 - 80y)/(60)`

x =
`(360 - 80.3)/(60)`

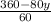
x = 2

To determine the cost:

cost = 42,000x + 51,000y

cost = 42000.2 + 51000.3

cost = 161400

To keep it in a minimun cost, it is needed 2 vessels of Type-A and 3 vessels of Type-B, to a cost of $161400