We have been given that a cone has a radius of 12 inches and slant height of 20 inches. We are asked to find the volume of the cone.
We will use volume of cone formula to solve our given problem.
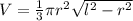 , where
, where
V = Volume of cone,
r = Radius,
l = Slant height.
Upon substituting our given values in volume formula, we will get:
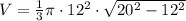
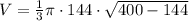
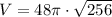
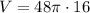
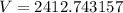
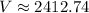
Therefore, the volume of the cone is approximately 2412.74 cubic inches.