Question

What is the value of g?

The image is of a circle with a tangent and a secant, which are intersecting at an angle 38 degrees. Measure of smaller arc cut by them is g degrees and that of bigger arc is 119 degrees.

A. 124
B. 43
C. 100
D. 86

Answer 1

We have been given a circle with a tangent and a secant, which are intersecting at an angle 38 degrees. We are asked to find the measure of arc g.

We can see that angle with 38 degrees measure is a secant tangent angle outside the circle.

We know that measure of an angle formed by intersection tangent and secant outside circle is half the difference of intercepted arcs.

`38^(\circ)=(1)/(2)(119^(\circ)-g^(\circ))`

`38^(\circ)\cdot 2=(1)/(2)\cdot 2(119^(\circ)-g^(\circ))`

`76^(\circ)=119^(\circ)-g^(\circ)`

`76^(\circ)+g^(\circ)=119^(\circ)-g^(\circ)+g^(\circ)`

`76^(\circ)+g^(\circ)=119^(\circ)`

`76^(\circ)-76^(\circ)+g^(\circ)=119^(\circ)-76^(\circ)`

`g^(\circ)=43^(\circ)`

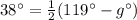
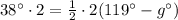
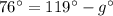
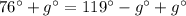
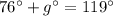
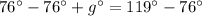
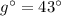
Therefore, the measure of smaller arc is 43 and option B is the correct choice.