Question

What is the volume of a hemisphere with radius 11? Round to the tenths.

Answer 1

2.786.23 cubic units

Explanation:

The volume of a full sphere is given by formula
`(4/3)\pi r^3`

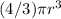
where r is the radius of full sphere.

Since hemisphere is half of full sphere , its volume is also half of full sphere which can be given by

`{(4/3)\pi r^3)/2}\\=> (2/3)\pi r^3`

_____________________________________

Given radius of hemisphere in the problem = 11

Thus volume of hemisphere is

=
`(2/3)\pi 11^3\\=>(2/3)\pi *1331\\=> 2/3*3.14*1331\\=>2,786.22667`

Thus, volume of hemisphere is 2.786.22667 cubic units

It is given that value should be rounded to tenths

So, volume of hemisphere is 2.786.23 cubic units rounded to tenth.