well, we know the endpoints of its diameter, so hmmm its center will be the midpoint of those endpoints.
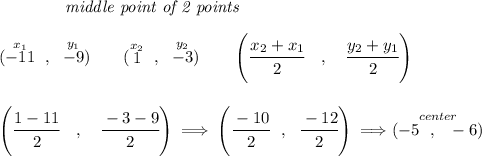
well, to get its radius, we can simply get the distance between both points and keeping in mind that the radius is half the diameter, we'll take half of that distance.
![~~~~~~~~~~~~\textit{distance between 2 points} \\\\ (\stackrel{x_1}{-11}~,~\stackrel{y_1}{-9})\qquad (\stackrel{x_2}{1}~,~\stackrel{y_2}{-3})\qquad \qquad d = √(( x_2- x_1)^2 + ( y_2- y_1)^2) \\\\\\ d=√([1 - (-11)]^2 + [-3 - (-9)]^2)\implies d=√((1+11)^2+(-3+9)^2) \\\\\\ d=√(12^2 + 6^2)\implies d=√(180)\implies d=6√(5)~\hfill \underset{half~that}{\stackrel{radius}{3√(5)}}](https://img.qammunity.org/2023/formulas/mathematics/high-school/qpmmjkqkuptrb7zy7finmf9ro05zkpequ0.png)