Question

Write the equation 6561 1/4= 9 in logarithmic form.

a.log 1 9/4= 6561
b. log6561 9 = 1/4
c.log9 6561 =1/4
d. log 1/4 6561 = 9

Answer 1

b. log6561(9) = 1/4

Explanation:

The relationship between the exponential form and the log form is ...

`b^n=x\\\log_b{(x)}=n`

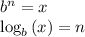
The exponent is the logarithm. The base is the value the exponent is being applied to.

Here, you have ...

`6561^{(1)/(4)}=9`

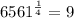
Comparing this to the form above, we see ...

b = 6561, n = 1/4, x = 9

so the log form of the equation is ...

`\boxed{log_6561((9))=(1)/(4)}`

This matches choice B.