Question

Las condiciones iniciales de un gas son 3000 cm3

, 1520 mm de Hg y27ºC, ¿Cuál será la nueva temperatura si el volumen se reduce a 2 L. y la presión

aumenta a 3 atm?

Answer 1

T'=92.70°C

Step-by-step explanation:

To find the temperature of the gas you use the equation for ideal gases:

`PV=nRT`

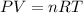
V: volume = 3000cm^3 = 3L

P: pressure = 1250mmHg; 1 mmHg = 0.001315 atm

n: number of moles

R: ideal gas constant = 0.082 atm.L/mol.K

T: temperature = 27°C = 300.15K

For the given values you firs calculate the number n of moles:

`n=(PV)/(RT)=((1520[0.001315atm])(3L))/((0.082(atm.L)/(mol.K))(300.15K))=0.200moles`

this values of moles must conserve when the other parameter change. Hence, you have V'=2L and P'=3atm. The new temperature is given by:

`T'=(P'V')/(nR)=((3atm)(2L))/((0.200\ moles)(0.082(atm.L)/(mol.K)))=365.85K=92.70\°C`

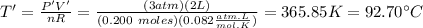
hence, T'=92.70°C