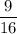Answer:
Explanation:
We are given that there are a total of 80 possibilities when a card a chosen from the box which has separate cards numbered from 1 to 80.
Case 1: Number of possible cases for even number to be chosen:
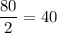
because from number 1 to 80, there will be a total of 40 even numbers
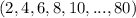
Case 2: Number of cases for an odd number below 10:
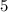 because below 10, the odd number possible are
because below 10, the odd number possible are
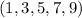
So, the total number of favorable cases as per the question = 45
Total Number of cases = 80

So, required probability is