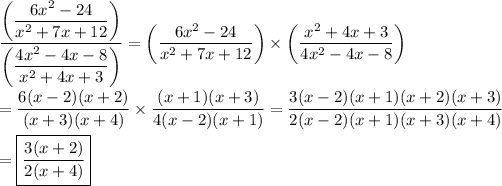Question

Help me out and please provide steps

Answer 1

3(x+2)/(2(x+4))

Explanation:

A compound fraction is simplified by rewriting it as a simple fraction, and reducing it to lowest terms by cancelling common factors from numerator and denominator. The division of one fraction by another is accomplished in the usual way: multiply the numerator by the inverse of the denominator.

__

`(\left((6x^2-24)/(x^2+7x+12)\right))/(\left((4x^2-4x-8)/(x^2+4x+3)\right))=\left((6x^2-24)/(x^2+7x+12)\right)*\left((x^2+4x+3)/(4x^2-4x-8)\right)\\\\=(6(x-2)(x+2))/((x+3)(x+4))*((x+1)(x+3))/(4(x-2)(x+1))=(3(x-2)(x+1)(x+2)(x+3))/(2(x-2)(x+1)(x+3)(x+4))\\\\=\boxed{(3(x+2))/(2(x+4))}`