Question

The maintenance department at the main campus of a large state university receives daily requests to replace fluorecent lightbulbs. The distribution of the number of daily requests is bell-shaped and has a mean of 55 and a standard deviation of 3. Using the 68-95-99.7 rule, what is the approximate percentage of lightbulb replacement requests numbering between 46 and 55?

Answer 1

The percentage of lightbulb replacement requests numbering between 46 and 55 is 49.87%.

Explanation:

Let the random variable X be defined as the number of daily requests to replace fluorescent lightbulbs.

The random variable X follows a Normal distribution with mean, μ = 55 and standard deviation, σ = 3.

To compute the probabilities of a Normally distributed random variable, we first need to convert the raw scores (i.e. X) to z-scores.

The formula to compute z-scores is:

`z=(X-\mu)/(\sigma)`

Compute the probability that the lightbulb replacement requests numbering between 46 and 55 as follows:

`P(46<X<55)=P((46-55)/(3)<(X-\mu)/(\sigma)<(55-55)/(3))`

`=P(-3<Z<0)\\\\=P(Z<0)-P(Z<-3)\\\\=0.50-0.0013\\\\=0.4987`

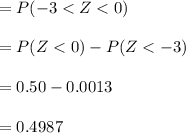
*Use a standard normal table.

The percentage is, 0.4987 × 100 = 49.87%.

Thus, the percentage of lightbulb replacement requests numbering between 46 and 55 is 49.87%.