Question

A desk lamp produced by The Luminar Company was found to be defective (D). There are three factories (A, B, C) where such desk lamps are manufactured. A Quality Control Manager (QCM) is responsible for investigating the source of found defects. This is what the QCM knows about the company's desk lamp production and the possible source of defects:

Factory % of total production
Probability of defective lamps
A
0.35 = P(A)
0.015 = P(D | A)
B
0.35 = P(B)
0.010 = P(D | B)
C
0.30 = P(C)
0.020 = P(D | C)
The QCM would like to answer the following question: If a randomly selected lamp is defective, what is the probability that the lamp was manufactured in factory C?

Answer 1

40.68% probability that the lamp was manufactured in factory C

Explanation:

Conditional probability formula:

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

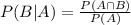
In which

P(B|A) is the probability of event B happening, given that A happened.

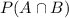`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

Noting that A and B here are general notations, not related to the factories in the question.

We have these following probabilities:

35% probability that a lamp is selected from factory A.

If a lamp is selected from factory A, 1.5% probability that it is defective.

35% probability that a lamp is selected from factory B.

If a lamp is selected from factory A, 1% probability that it is defective.

30% probability that a lamp is selected from factory C.

If a lamp is selected from factory A, 2% probability that it is defective.

If a randomly selected lamp is defective, what is the probability that the lamp was manufactured in factory C?

Event A: Defective lamp

Event B: From factory C.

`P(A) = 0.35*0.015 + 0.35*0.01 + 0.3*0.02 = 0.01475`

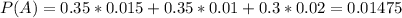
Intersection:

30% probability that a lamp is selected from factory C.

If a lamp is selected from factory A, 2% probability that it is defective.

Then

`P(A \cap B) = 0.3*0.02 = 0.006`

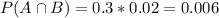
Finally:

`P(B|A) = (P(A \cap B))/(P(A)) = (0.006)/(0.01475) = 0.4068`

40.68% probability that the lamp was manufactured in factory C