Question

g The average teachers salary in north Dakota is $37,764. Assume a normal distribution with LaTeX: \sigma=\text{5100}σ = 5100. a) For a sample of 75 teachers, what is the probability that the mean is greater than $38,000? Present your answer in 4 decimal places. b) What is the probability that a randomly selected teacher's salary is grater than $45,000? Present your answer in three decimal places. Present your answers as: a:___,b:___

Answer 1

(a) Probability that the mean is greater than $38,000 is 0.3446.

(b) Probability that a randomly selected teacher's salary is grater than $45,000 is 0.078.

Explanation:

We are given that the average teachers salary in north Dakota is $37,764. Assume a normal distribution with sigma (σ) = 5100.

(a) A sample of 75 teachers is taken.

Let
`\bar X` = sample mean salary

The z score probability distribution for sample mean is given by;

Z =
`(\bar X-\mu)/((\sigma)/(√(n) ) )` ~ N(0,1)

where,
`\mu` = population mean salary = $37,764

`\sigma` = standard deviation = $5,100

n = sample of teachers = 75

Now, probability that the mean is greater than $38,000 is given by = P(
`\bar X` > $38,000)

P(
`\bar X` > $38,000) = P(
`(\bar X-\mu)/((\sigma)/(√(n) ) )` >
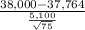`(38,000-37,764)/((5,100)/(√(75) ) )` ) = P(Z > 0.40) = 1 - P(Z < 0.40)

= 1 - 0.6554 = 0.3446

The above probability is calculated by looking at the value of x = 0.40 in the z table which has an area of 0.6554.

(b) Let X = a randomly selected teacher's salary

The z score probability distribution for normal distribution is given by;

Z =
`( X-\mu)/(\sigma)` ~ N(0,1)

where,
`\mu` = population mean salary = $37,764

`\sigma` = standard deviation = $5,100

Now, probability that a randomly selected teacher's salary is grater than $45,000 is given by = P(X > $45,000)

P(X > $45,000) = P(
`( X-\mu)/(\sigma)` >
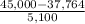`(45,000-37,764)/(5,100) }` ) = P(Z > 1.42) = 1 - P(Z < 1.42)

= 1 - 0.9222 = 0.078

The above probability is calculated by looking at the value of x = 1.42 in the z table which has an area of 0.9222.