Question

"An uncharged 30.0-µF capacitor is connected in series with a 25.0-Ω resistor, a DC battery, and an open switch. The battery has an internal resistance of 10.0 Ω and the open-circuit voltage across its terminals is 50.0 V. The leads have no appreciable resistance. At time t = 0, the switch is suddenly closed." "When does the maximum current occur?"

Answer 1

1.04x
`10^(-3)` s

Step-by-step explanation:

->The maximum current through the resistor is

`I_(max)` = V/R = V/
`Re^(-t/RC)`= V/R×
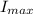`e^(0)` = V/R

Voltage 'V'=50V

Effective resistance 'R'= 25.0-Ω+ 10.0 Ω= 35.0 Ω

Therefore,
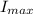`I_(max)`=50/35=> 1.43 A

->The maximum charge can be determined by

Q = CV

where,

Capacitance of the capacitor 'C' = 30.0µF = 30×10-⁶F

Therefore,

Q=30×10-⁶ x 50=>1.5 x
`10^(-3)`

In order to find that when does the maximum current occur, the time taken given the quantity of charge and the electric current is:

t= Q / I=> 1.5 x
`10^(-3)`/ 1.43

t= 1.04x
`10^(-3)` s