Step-by-step explanation:
Let l is the length of the wire. such that, side of square is l/4. Area of square loop is,
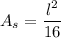
Radius of the circular loop,
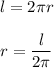
Area of the circular loop,

Torque in magnetic field is given by :
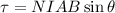
It is clear that,
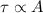
So,

So, the ratio of maximum torque on the square loop to the maximum torque on the circular loop is
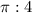 .
.