Question

You wish to test the following claim (Ha) at a significance level of α = 0.01.

H0: μ1 = μ2
Ha: μ1 /= μ2
You believe both populations are normally distributed, but you do not know the standard deviations for either. And you have no reason to believe the variances of the two populations are equal.
You obtain a sample of size n1 = 22 with a mean of x1^- = 56.2 and a standard deviation of s1 = 18.2 from the first population.
You obtain a sample of size n2 = 11 with a mean of x2^- = 64.2 and a standard deviation of s2 = 13.9 from the second population.
a) What is the test statistic for this sample?
b) What is the p-value for this sample?

Answer 1

a)
`t=\frac{(56.2-64.2)-0}{\sqrt{(18.2^2)/(22)+(13.9^2)/(11)}}}=-1.40`

b)
`p_v =2*P(t_(31)<-1.4)=0.171`

Explanation:

Information given

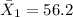`\bar X_(1)=56.2` represent the mean for sample 1

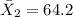`\bar X_(2)=64.2` represent the mean for sample 2

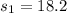`s_(1)=18.2` represent the sample standard deviation for 1

`s_(2)=13.9` represent the sample standard deviation for 2

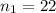`n_(1)=22` sample size for the group 2

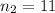`n_(2)=11` sample size for the group 2

t would represent the statistic (variable of interest)

System of hypothesis

We need to conduct a hypothesis in order to check if the true means are different, the system of hypothesis would be:

Null hypothesis:
`\mu_(1)-\mu_(2)=0`

Alternative hypothesis:
`\mu_(1) - \mu_(2)\\eq 0`

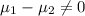
The statistic is given by:

`t=\frac{(\bar X_(1)-\bar X_(2))-\Delta}{\sqrt{(s^2_(1))/(n_(1))+(s^2_(2))/(n_(2))}}` (1)

The degrees of freedom are given by:

`df=n_1 +n_2 -2=22+11-2=31`

Part a: Statisitc

Replacing into the formula we got:

`t=\frac{(56.2-64.2)-0}{\sqrt{(18.2^2)/(22)+(13.9^2)/(11)}}}=-1.40`

Part b: P value

The p value on this case would be:

`p_v =2*P(t_(31)<-1.4)=0.171`