Question

Use the given degree of confidence and sample data to construct a confidence interval for the population mean mu. Assume that the population has a normal distribution. Thirty randomly selected students took the calculus final. If the sample mean was 95 and the standard deviation was​ 6.6, construct a​ 99% confidence interval for the mean score of all students.

Answer 1

`95-2.76(6.6)/(√(30))=91.674`

`95+2.76(6.6)/(√(30))=98.326`

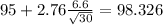
We can say at 99% confidence that the true mean is between (91.674;98.326)

Explanation:

Data given

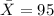`\bar X=95` represent the sample mean

`\mu` population mean (variable of interest)

s=6.6 represent the sample standard deviation

n=30 represent the sample size

The confidence interval for the mean is given by the following formula:

`\bar X \pm t_(\alpha/2)(s)/(√(n))` (1)

We need to find the critical value
`t_(\alpha/2)` and we need to find first the degrees of freedom, given by:

`df=n-1=30-1=29`

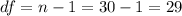
Since the Confidence is 0.99 or 99%, the value of
`\alpha=0.01` and
`\alpha/2 =0.005`, and we can use excel, a calculator or a table to find the critical value. The excel command would be: "=-T.INV(0.005,29)".And we see that
`t_(\alpha/2)=2.76`

And replacing we got:

`95-2.76(6.6)/(√(30))=91.674`

`95+2.76(6.6)/(√(30))=98.326`

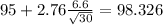
We can say at 99% confidence that the true mean is between (91.674;98.326)