Answer:
At p₀ = 0.982 the manufacturer's claim would not be rejected.
Explanation:
The manufacturer wishes to make a claim about the percentage of non-defective CD players and is prepared to exaggerate.
Let the claim made by him be denoted as, p₀.
The hypothesis to test this claim is defined as:
H₀: The proportion of defective is p₀, i.e. p = p₀.
Hₐ: The proportion of defective is less than p₀, i.e. p < p₀.
The significance level of the test is, α = 0.05.
The information provided is:
n = 100
 = 0.96
= 0.96
The test statistic is:
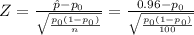
Decision rule:
If the test statistic value is less than the critical value of z, i.e. Z₀.₀₅ = -1.645 (because of the left tail), then the null hypothesis will be rejected. and vice versa.
So, to reject H₀ Z ≤ Z₀.₀₅.
Use hit and trial method.
At p₀ = 0.97 the value of Z is:

At p₀ = 0.98 the value of Z is:
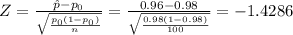
At p₀ = 0.981 the value of Z is:
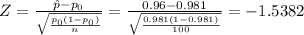
At p₀ = 0.982 the value of Z is:
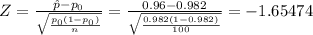
At p₀ = 0.982 the value of Z is -1.65474.
Z = -1.65474 > Z₀.₀₅ = -1.645
Thus, at p₀ = 0.982 the manufacturer's claim would not be rejected.