Question

The first term of a geometric progression is 3 and the common ratio is 2 a)Write down the forth term of the progression b)write down the sixth term of the progression

Answer 1

a) Fourth term of the progression is 24

b) Sixth term of the progression is 96

Explanation:

Formula for geometric sequence is,

`a_(n)=ar^(n-1)`

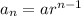
where
`a_(n)=n^(th) term\:of\:sequence`

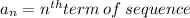
a = first term of sequence

r = common ratio

Given that first term of GP is 3. So, a = 3. Also common ratio is 2. So, r = 2.

a) To find fourth term of progression that is,
`a_(4)`.

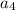Substituting the values in given formula,

`a_(4)=3\left(2^(4-1)\right)`

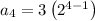
Simplifying,

`a_(4)=3\left(2^(3)\right)`

`a_(4)=3\left(8\right)`

`a_(4)=24`

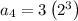
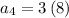
Therefore, the fourth term of progression is 24

b) To find sixth term of progression that is,
`a_(6)`.

Substituting the values in given formula,

`a_(6)=3\left(2^(6-1)\right)`

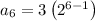
Simplifying,

`a_(6)=3\left(2^(5)\right)`

`a_(6)=3\left(32\right)`

`a_(6)=96`

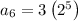
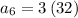
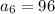
Therefore, the sixth term of progression is 96