Answer:
The initial investment was of $3,610.
Explanation:
The continuous interest formula is:
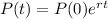
In which P(t) is the amount of money after t years, P(0) is the initial amount invested and r is the fixed interest rate.
6.79% compounded continuously.
This means that
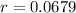
After 20 years, the balance reaches $14,037.16.
This means that
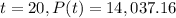
What was the amount of the initial investment?
This is P(0).
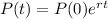

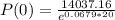
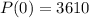
The initial investment was of $3,610.