Question

The common ratio in a geometric series is 0.5 and the first term is 256. Find the sum of the first 6 terms in the series

Answer 1

The sum of the first 6 terms of the series is 504.

Explanation:

Given that,

Common ratio in a geometric series is, r = 0.5

First term of the series, a = 256

We need to find the sum of the first 6 terms in the series. If a and r area the first term and common ratio of a series, then the series becomes:

`a,ar^1,ar^2,ar^3......`

The sum of n terms of a GP is given by :

`S_n=(a(1-r^n))/(1-r)`

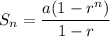
Here, n = 6

`S_n=(256* (1-(0.5)^6))/(1-0.5)\\\\S_n=504`

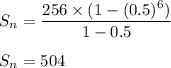
So, the sum of the first 6 terms of the series is 504.