Answer:
The maximum volume is 122.73 mL
Step-by-step explanation:
125 mL of butter that is 0.360 M in CH₃COOH and CH₃COO⁻.
pKa of acetic acid = 4.76
Using Henderson Hasselbalch equation.
pH =pKa + log
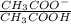
= 4.76 +
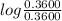
= 4.76
Assuming the pH of the buffer changes by a unit of 1 , then it will lose its' buffering capacity.
When HCl is added , it reacts with CH₃COO⁻ to give CH₃COOH.
However, [CH₃COOH] increases and the log term results in a negative value.
Let assume, the new pH is less than 4.76
Let say 3.76; calculating the concentration when the pH is 3.76; we have
3.76 = 4.76 + log
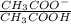
log
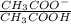 = 4.76 - 3.76
= 4.76 - 3.76
log
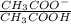 = 1.00
= 1.00
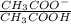 = 0.1
= 0.1
Let number of moles of acid be x (i.e change in moles be x); &
moles of acetic acid and conjugate base present be = Molarity × Volume
= 0.360 M × 125 mL
= 45 mmol
replacing initial concentrations and change in the above expression; we have;
![([CH_3COO^-])/([CH_3COOH]) = 0.1](https://img.qammunity.org/2021/formulas/chemistry/college/1882dvuhkavielz5mu1s5w2q5dkmbs0wvo.png)
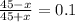
0.1(45 + x) = 45 - x
4.5 + 0.1 x = 45 -x
0.1 x + x = 45 -4.5
1.1 x = 40.5
x = 40.5/1.1
x = 36.82
So, moles of acid added = 36.82 mmol
Molarity = 0.300 M
So, volume of acid =
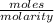 =
=
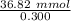
= 122.73 mL