Question

Which is the graph of linear inequality x – 2y ≥ –12?

On a coordinate plane, a dashed straight line with positive slope goes through (negative 8, 2) and (0, 6). Everything to the right of the line is shaded.
On a coordinate plane, a solid straight line with positive slope goes through (negative 8, 2) and (0, 6). Everything to the left of the line is shaded.
On a coordinate plane, a dashed straight line with negative slope goes through (negative 6, 6) and (0, negative 6). Everything to the right of the line is shaded.
On a coordinate plane, a solid straight line with positive slope goes through (negative 8, 2) and (0, 6). Everything to the right of the line is shaded.

Answer 1

Answer: On a coordinate plane, a solid straight line with positive slope goes through (negative 8, 2) and (0, 6). Everything to the right of the line is shaded.

Step-by-step explanation:

Answer 2

The correct option is: On a coordinate plane, a solid straight line with positive slope goes through
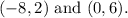`\((-8, 2)\) and \((0, 6)\).` Everything to the right of the line is shaded.

The correct graph for the linear inequality
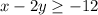`\(x - 2y \geq -12\)` is:

On a coordinate plane, a solid straight line with positive slope goes through
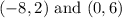`\((-8, 2)\) and \((0, 6)\)`. Everything to the right of the line is shaded.

So, the correct option is:

On a coordinate plane, a solid straight line with positive slope goes through
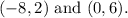`\((-8, 2)\) and \((0, 6)\).` Everything to the right of the line is shaded.