Question

A local car dealer claims that 25% of all cars in San Francisco are blue. You take a random sample of 600 cars in San Francisco and find that 141 are blue. Conduct a hypothesis test to see if you can reject the dealer's claim with a significance level of 0.05. Include all ten steps of the hypothesis testing process. Write your answer on a piece of paper and upload a photo or scan, or else type your answer into a electronic document and upload that document. In order to get full credit you must

Answer 1

No, we can't reject the dealer's claim with a significance level of 0.05.

Explanation:

We are given that a local car dealer claims that 25% of all cars in San Francisco are blue.

You take a random sample of 600 cars in San Francisco and find that 141 are blue.

Let p = proportion of all cars in San Francisco who are blue

SO, Null Hypothesis,
`H_0` : p = 25% {means that 25% of all cars in San Francisco are blue}

Alternate Hypothesis,
`H_A` : p
`\\eq` 25% {means that % of all cars in San Francisco who are blue is different from 25%}

The test statistics that will be used here is One-sample z proportion statistics;

T.S. =
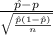`\frac{\hat p-p}{{\sqrt{(\hat p(1-\hat p))/(n) } } } }` ~ N(0,1)

where,
`\hat p` = sample proportion of 600 cars in San Francisco who are blue =
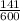`(141)/(600)` = 0.235

n = sample of cars = 600

So, test statistics =
`\frac{0.235-0.25}{{\sqrt{(0.235(1-0.235))/(600) } } } }`

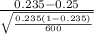
= -0.866

Now at 0.05 significance level, the z table gives critical values of -1.96 and 1.96 for two-tailed test. Since our test statistics lies within the range of critical values of z so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region due to which we fail to reject our null hypothesis.

Therefore, we conclude that 25% of all cars in San Francisco are blue which means the dealer's claim was correct.