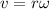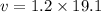Question

solid disk at rest starts to spin. The angular acceleration is constant.After 3 revolutions the wheel is spinning at 6radsec. Find the linear velocity andacceleration of a point on the disk 1.2 meters away from the center (axis) at20 seconds.

Answer 1

Step-by-step explanation:

Given,

Angular displacement, θ = 3 rev

= 3 x 2π = 6π

initial angular speed, ω = 0 rad/s

Angular speed when disk is revolved to 3 revolution = 6 rad/s

radius, r = 1.2 m

time, t = 20 s

Calculating angular acceleration

`\omega^2 = \omega_0^2 +2 \alpha \theta`

`(6)^2= 0 +2 \alpha* (6\pi)`

`\alpha = 0.955\ rad/s^2`

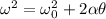
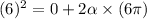
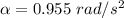
Acceleration of the disk

`a = \alpha R`

`a =0.955* 1.2 = 1.146\ m/s^2`

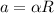
Now for linear velocity calculation

`\omega = \omega_0 + \alpha t`

`\omega =0 +0.955* 20`

`\omega = 19.1\ rad/s`

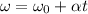
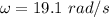
Linear velocity,

`v = r \omega`

`v = 1.2 * 19.1`

`v = 22.92\ m/s`