Question

Sample size allocation. Suppose we are testing the hypotheses

H0:μ1 = μ2
H1:μ1 not equal μ2

where σ12 and σ22 are known. Resources are limited, and consequently the total sample size n1 + n2 = N. How should we allocate the N observations between the two populations to obtain the most powerful test?

Answer 1

The test statistic

`Z = \frac{x^(-) _(1)-x^(-) _(2) }{\sqrt{('σ^2_(1) )/(n_(1) ) +('σ^2_(2) ')/(n_(2) ) } }`

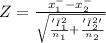
Explanation:

Explanation:-

Let x₁⁻ be the mean of the sample of size n₁ from a population mean μ₁ and standard deviation 'σ₁

Let x₂⁻ be the mean of the sample of size n₂ from a population mean μ₂ and standard deviation 'σ₂'

Null hypothesis : H0:μ₁ = μ₂

Alternative hypothesis : H1:μ₁ ≠μ₂

To test whether there is any significant difference between x₁⁻ and x₂⁻ we have use test statistic

`Z = \frac{x^(-) _(1)-x^(-) _(2) }{\sqrt{('σ^2_(1) )/(n_(1) ) +('σ^2_(2) ')/(n_(2) ) } }`

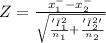
Here

• x₁⁻ be the mean of the first sample
• x₂⁻ be the mean of the second sample
• 'σ₁' be standard deviation first population.

• 'σ₂' be standard deviation second population.