Question

A ray of light, traveling through air, is incident on a smooth transparent liquid surface at an angle of 13 degrees with respect to the normal of the surface. What is the refraction angle of the light in the liquid if the index of refraction of the liquid is 1.54? Return the angle in degrees and rounded to 2 decimal places.

Answer 1

The refraction angle of the light in the liquid is 8.40 degrees.

Step-by-step explanation:

Given:

A ray of light passing through air to liquid.

Air is medium 1 and liquid is medium 2.

Angle of incidence
`(\theta_1)` = 13°

Refractive index,
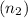`(n_2)` = 1.54

We have to find the angle of refraction:

Let the angle of refraction be "
`\theta_2`" .

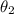Formula to be used:

⇒
`n_1* sin(\theta_1) =n_2* sin(\theta_2)`

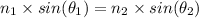
Note:

Index of refraction of air
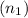`(n_1)` = 1

Accordingly:

Using Snell's law and plugging the values.

⇒
`n_1* sin(\theta_1) =n_2* sin(\theta_2)`

⇒
`1* sin(13) =1.54* sin(\theta_2)`

⇒
`(1* sin(13))/(1.54) = sin(\theta_2)`

⇒
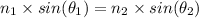
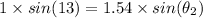
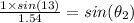
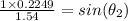`(1* 0.2249)/(1.54) = sin(\theta_2)` ...sin(13) =0.2249

⇒
`\theta_2=sin^-^1((0.2249)/(1.54))`

⇒
`\theta_2=sin^-^1(0.145)`

⇒
`\theta_2=8.3974` degrees.

⇒
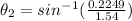
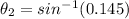
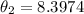`\theta_2 = 8.40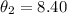` degrees ...Rounded to 2 decimal place.

The refraction angle of the light in the liquid is 8.40 degrees.