Question

1) Aladder is placed against the wall of a building such that the bottom of the ladder is 3 ft from the bottom of the wall. If the ladder forms a 14 degree angle with the building, how high up the wall does the ladder reach?​

Answer 1

0.75 feet

Explanation:

Let's picture a right triangle, where :

• AB = distance b/w bottom of ladder and bottom of wall = 3 feet
• AC = length of ladder [what we need to find!]
• BC = distance b/w top of building and bottom of ladder

Let the height of the ladder be x.

• Then, taking the ratio b/w the adjacent and opposite sides...
• ⇒ tan 14° = 0.249
• ⇒ tan 14° = x/3
• ⇒ x/3 = 0.249
• ⇒ x = 0.249 x 3
• ⇒ x = 0.747 ≅ 0.75 feet

Answer 2

0.748 ft (nearest thousandth)

Explanation:

Use the tan trig ratio:

`\tan(\theta)=(O)/(A)`

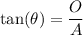
where:

• 
  `\theta` is the angle
• O is the side opposite the angle
• A is the side adjacent the angle

Given:

• 
  `\theta` = 14°
• O = x
• A = 3 ft

`\implies \tan(14)=(x)/(3)`

`\implies x=3\tan(14)`

`\implies x=0.7479840085...`

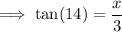
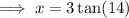
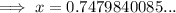
Therefore, the ladder reaches up the wall 0.748 ft (nearest thousandth)